The Similarity Principle and Its Application

Shu-Kun Lin

Molecular Diversity Preservation International (MDPI), Matthaeusstrasse 11, CH-4057 Basel, Switzerland (e-mail: [email protected], http://www.mdpi.org/lin)

The Similarity Principle:

If all the other conditions remain constant, the higher the similarity among the components of an ensemble (or a considered system) is, the higher value of entropy of the mixture (for fluid phases) or the assemblage (for a static structure or a system of solid phase) or any other structure (such as an ensemble of quantum states in quantum mechanics) will be, the more stable the mixture or the assemblage will be, and the more spontaneous the process leading to such a mixture or assemblage will be.
This theory [1] is very useful for characterizing structural stability and process spontaneity. For example, it is applied to the phase separation as a simple rule: If one wants to mix substances, increase their similarity (of relevant properties); if one plans to separate the substances as phases, reduce their similarity! Then, the desirable processes of mixing or separation will happen spontaneously. In chemistry and physics, by changing temperature and pressure, one can control the similarity and in turn, the system will go to the desired direction (e.g., phase separation or homogeneous mixture formation). Higher temperature and pressure may lead to higher similarity. This theory is important in understanding molecular recognition, self-organization, molecular assembling and molecular replication.

Figure 1. Correlation of the entropy of mixing with similarity. The Gibbs paradox statement (see http://www.mdpi.org/lin/entropy/gibbs-paradox.htm), which has been regarded as a very fundamental assumption in statistical mechanics, says that the entropy of mixing or assembling to form solid assemblages, liquid and gas mixtures or any other analogous assemblages such as quantum states, decreases discontinuously (Fig.1a) with the increase in the property similarity of the composing individuals. Some authors revised the Gibbs paradox statement and argued that the entropy of mixing decreases continuously with the increase in the property similarity of the individual components (Fig.1b). This statement has been discarded and a new theory constructed: entropy of mixing or assembling increases continuously (Fig.1c) with the increase in the similarity [1]. Similarity Z can be easily understood when two items A and B are compared: if A and B are distinguishable (minimal similarity), Z=0. If they are indistinguishable (maximal similarity), Z=1.

Experimental Facts: (a) Phase separation. Different substances do not mix but spontaneously separate because the indistinguishable substances are the most spontaneously miscible ones. As a consequence of the most spontaneously mixing the most similar (indistinguishable) substances, different substances separate. (b) Chemical bond formation or Pauling's resonance (mixing of the quantum states). (c) Information registration process () is a process of assembling different species. (d) All symmetry-breaking phenomena also indicate that the assembling of indistinguishable subsystems is the most spontaneous process leading to the most stable state.

Theoretical Arguments: (a) From the well-known inequality

                                        (1)
and the general entropy expression
                                        (2)
the condition for the maximum entropy must be the indistinguishability among the w components. (b) The Gibbs paradox statement of entropy of mixing contradicts the entropy additivity principle suggested by Gibbs himself. The Gibbs entropy additivity principle should be in the same form as the Dalton pressure additivity for ideal gases. Gibbs paradox statement will violate the basic definition that entropy is an extensive variable. (c) An ideal gas is defined as a gas consisting of independent particles. However, the inclusion of the term  in the Sackur-Tetrode entropy formula according to Gibbs implies that the N particles in consideration are not independent.

From (1),

                                         (3)
defines a similarity index, and entropy increases continuously with the property similarity of the w microstates or w subsystems. The similarity depends directly on the similarity among the considered components.

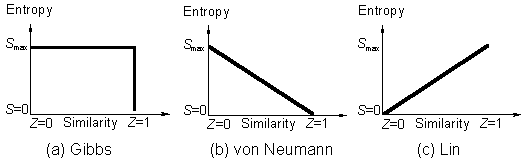
Entropy also increases with the symmetry. Given two systems which are identical in material contents, energies, and all the other properties except their symmetries which are the only difference. Which system would be more stable, the one with higher symmetry or the one with lower symmetry? For a solid phase, is the crystalline structure or the non-crystalline structure more stable?

In answering this structural relative stability question, the entropy S is a pertinent thermodynamic function to consider. However, according to "higher symmetry-higher orderliness-less entropy-less stability" relation in all statistical mechanics (see references cited in ref. [1a]), such as the treatment of the Ising model of symmetry breaking problems, higher symmetry in a system would imply a lower value of S, and the answer would be that the less symmetrical static structure is more stable. This is incorrect and does not conform to the experimental observation (also read my comments in [3]).

Curie's symmetry principle says "the symmetry group of the cause is a subgroup of the symmetry group of the effect" [2]. Semantically, the Greek word symmetry means same measure or indistinguishability measure.The Curie-Rosen symmetry principle [2] can be proved [1h] by the similarity principle and it is correct.

A logarithmic relation (eq 4) has been established [1a] which conforms to Curie's symmetry principle [2]. For a system of w microstates,

                                                         (4)
(k is positive) and the apparent symmetry number or apparent indistinguishability number is
                                                        (5)
where  is the probability of the ith microstate. In conclusion, a higher value of entropy of any system correlates to a higher symmetry and a higher stability, whether they are dynamic systems or static structures [1a,1h]. 

References and Notes:

1. (a) Lin, S.-K. Correlation of entropy with similarity and symmetry. J. Chem. Inf. Comp. Sci. 1996, 36, 367-376. (b) Lin, S.-K. Gibbs paradox of entropy of mixing: experimental facts, its rejection and the theoretical consequences. Electronic Journal of Theoretical Chemistry. 1996, 1, 135-150; (c) Lin, S. -K. Molecular diversity assessment: Logarithmic relations of information and species diversity and logarithmic relations of entropy and indistinguishability after rejection of Gibbs paradox of entropy of mixing. Molecules 1996, 1, 57-67. (d) Lin, S.-K. Understanding structural stability and process spontaneity based on the rejection of the Gibbs paradox of entropy of mixing. Theochem–J. Mol. Struc. 1997, 398, 145-153. (e) Lin, S.-K. Symmetry breaking problem resolved (American Physical Society Meeting, Kansas City, MO, March 17-21, 1997). Bull. Am. Phys. Soc. 1997, 42, 679. (f) Lin, S.-K. The nature of the chemical process. A new information theory. The 36th IUPAC Congress, Geneva, Switzerland, August 17-22, 1997. Chimia 1997, 51, 515. (g) Lin, S.-K. Similarity rule and complementarity rule, Chimia 1999, 53, 383. (http://www.mdpi.org/lin/basel99.htm) (h) Lin, S. -K. The Nature of the Chemical Process. 1. Symmetry Evolution –Revised Information Theory, Similarity Principle and Ugly Symmetry. Int. J. Mol. Sci. 2001, 2, 10-39. (i) Lin, S.-K. Gibbs Paradox and the Concepts of Information, Symmetry, Similarity and Their Relationship. Entropy 2008, 10, 1-5. DOI: 10.3390/entropy-e10010001. arXiv:0803.2571. (j) Lin, S.-K. Gibbs Paradox and Similarity Principle. Paper presented at MaxEnt2008. 2008, arXiv:0807.4314v1 [physics.gen-ph].

2. (a) Rosen, J. Symmetry in Science; Springer: New York, 1995. See a book review. (b) Rosen, J. The Symmetry Principle. Entropy, 2005, 7(4), 308-313.

3. Lin, S. -K. Lecture entitled "Ugly Symmetry" at many universities and international conferences. E.g., Ugly Symmetry, at the 218th ACS National Meeting August 22-26, 1999, New Orleans, Louisiana. (http://www.mdpi.org/lin/uglysym1.htm). A longer version: link.

http://www.mdpi.org/lin/similarity/similarity.htm
Return to http://www.mdpi.org/lin/
First time uploaded: 1 January 1998 / Updated: 2 September 2008